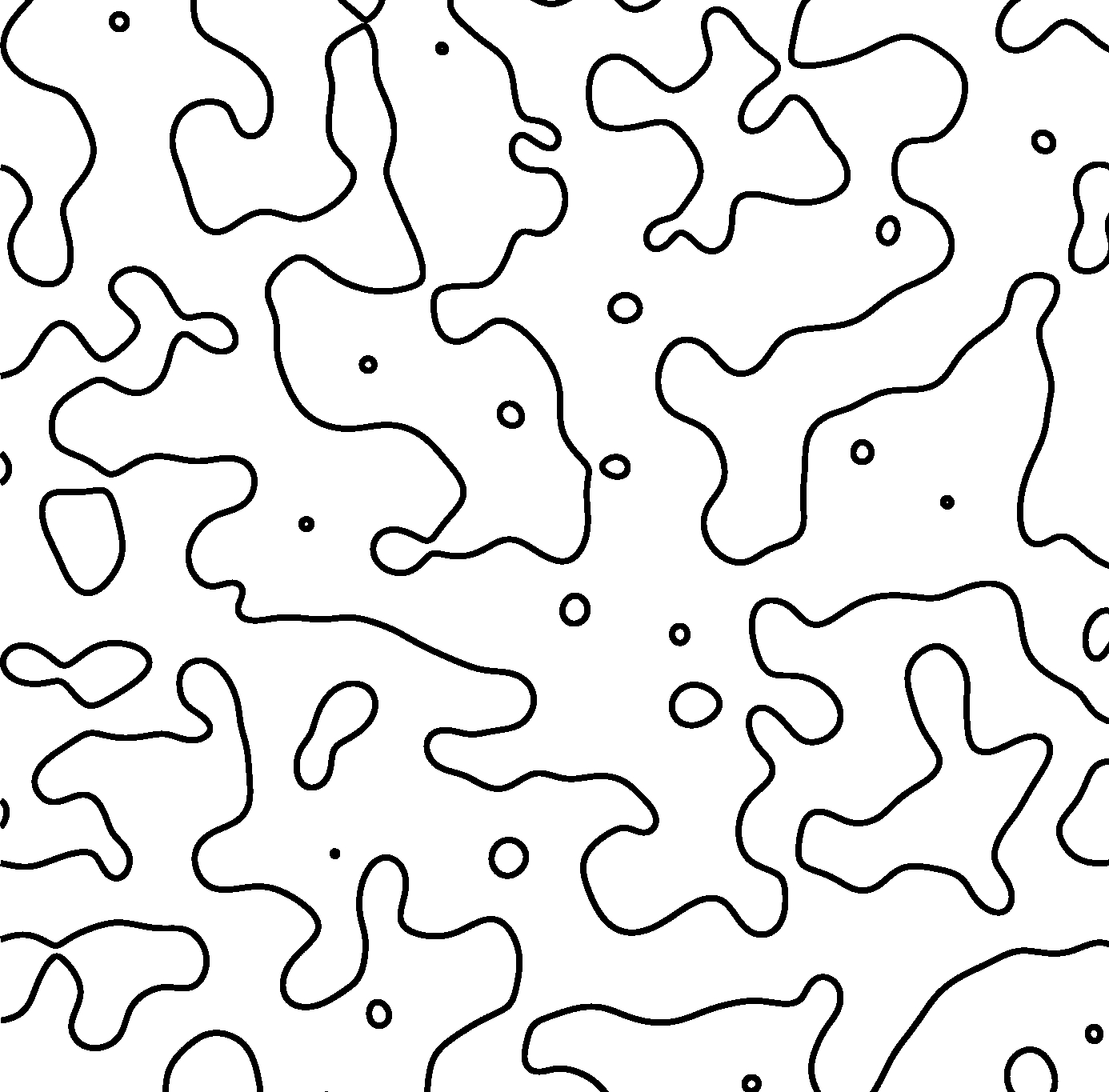
Study the typical geometry and topology
of a random lemniscate.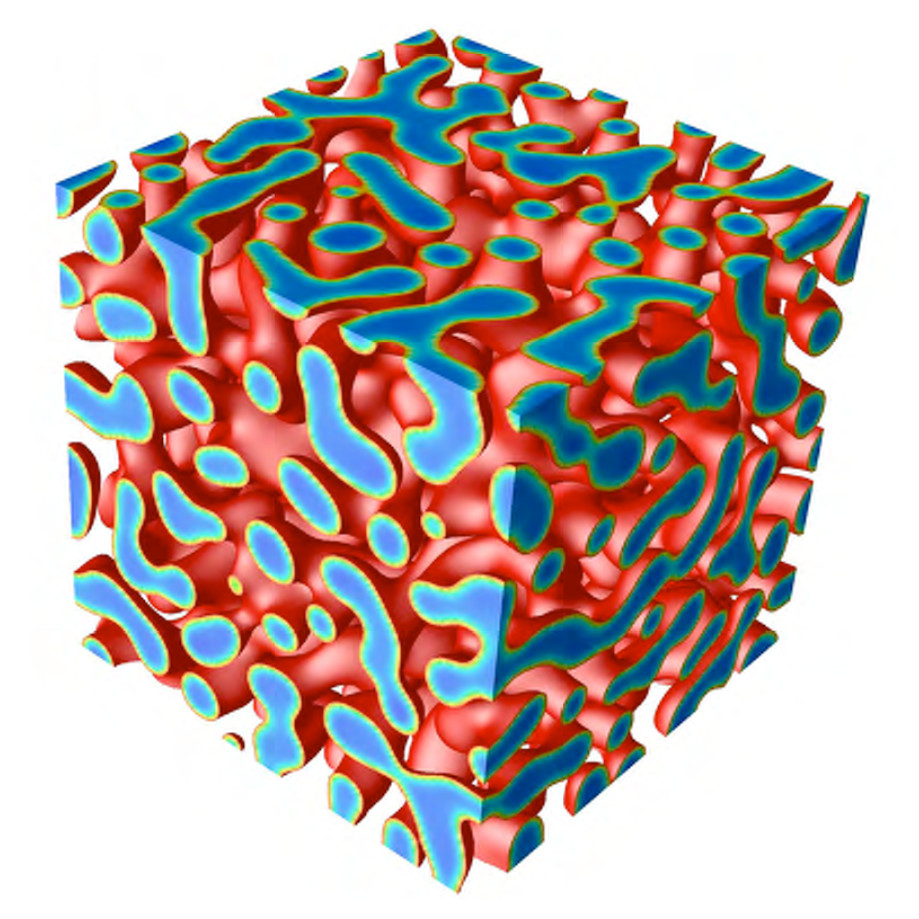

Study the Betti numbers of a random manifold.

Study the average number of real solutions to
problems in enumerative geometry, e.g.,
how many lines are there on a random cubic?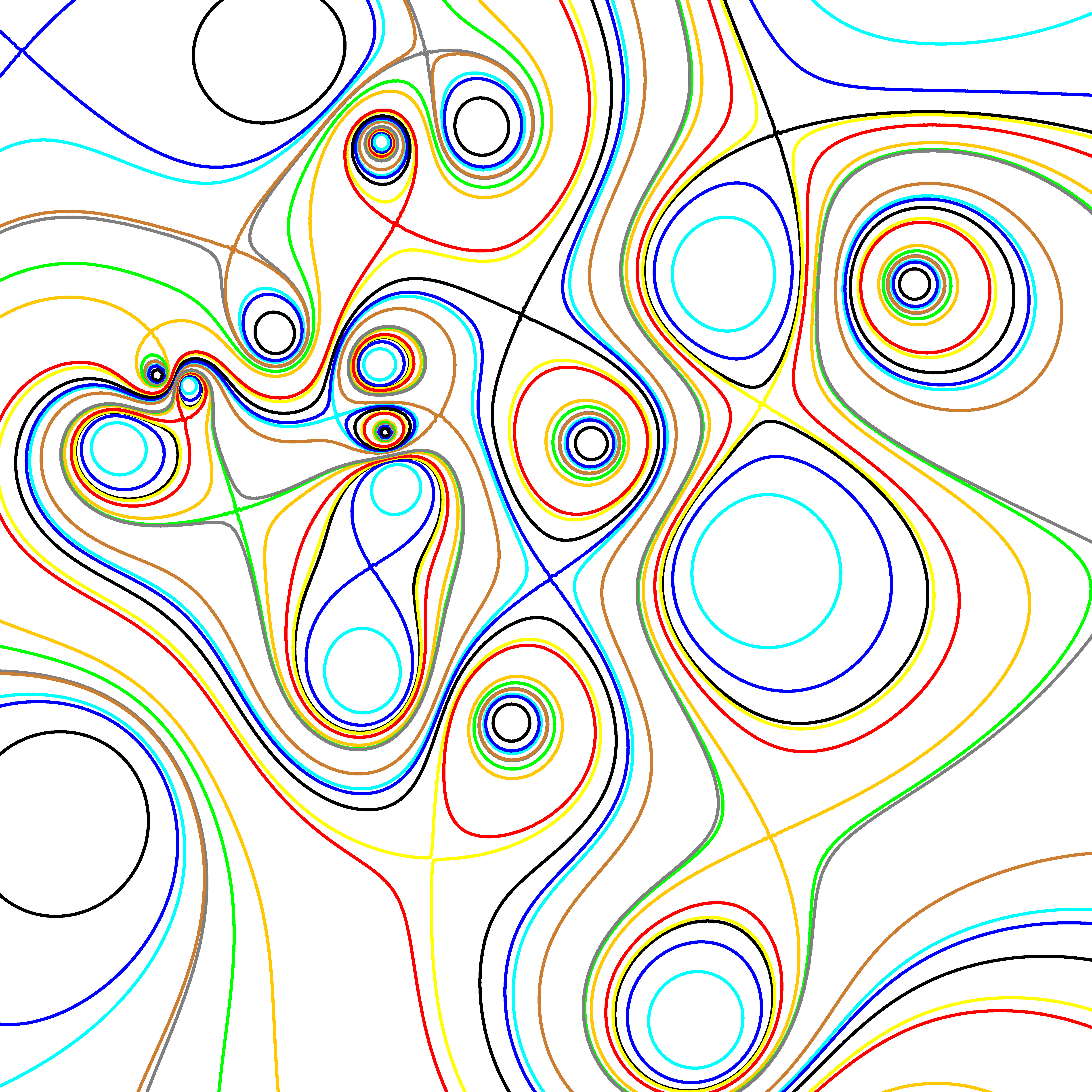

What can we say about the structure of the
Reeb graph of a random landscape?

How many cusps are there on a random caustic?

• Motivation:

  Motivation for studying the topology of random manifolds comes from several sources.

• First, taking a random viewpoint on Hilbert's sixteenth problem brings a much-needed broad perspective on the topology of real algebraic varieties. This is not only for the sake of pure mathematics, as real algebraic geometry forms a basis for computational geometry, considered by many to be the most urgent frontier of algorithms. Any future development of average case analysis of algorithms in computational geometry will need to build on a body of probabilistic results in the setting of random real algebraic manifolds.

• A second source of motivation comes from conjectures in quantum chaos concerning the nodal sets of random eigenfunctions. This also relates to real algebraic geometry as eigenfunctions on certain manifolds such as spheres and tori can be represented algebraically.

• Third, some model problems of statistical physics, such as those of spin glasses, concern topological features of random potential energy landscapes.

• Fourth, random manifolds provide natural smooth/analytic settings for investigating phase transitions, such as percolation of giant components, that have primarily been studied in discrete lattice models.

• Fifth, the recent successes of Topological Data Analysis prompts further investigation of persistent homology in the context of random manifolds. Studying the outcome for parameter spaces of generative models is important for establishing baselines and eventually building a theory of Statistical Inference within Topological Data Analysis.

  Problems:

• Problem 1: Study the connected components of a random curve. How they embedded in the plane, and what is the distribution of lengths of components?

• Problem 2: Study the Betti numbers of a random manifold.

• Problem 3: Study enumerative geometry from the random viewpoint. For example, how many lines are on a random cubic?

• Problem 4: Study the family of level sets of a random landscape. How are the singular levels embedded in the plane?

• Problem 5: Study the cusps on a caustic (set of critical values) associated to a random harmonic mapping.